Biology in UFT1
Unified Fields Theory 1
-
Phil Seawolf / Philip Self
*NOTE: All images, artwork and audio are made on my Mac with Pages (all the art), Garage Band (music) and other Mac Applications Etc... and are all 100% originals. My voice is my own and I have fun with accent and personality. Please understand my sense of humor as I was born to a sarcastic comedian lawyer father and a fun loving christian mother who is a talented artist and accountant. Go figure. We try and fire on both sides of the brain in our family. Biography Scroll down for details or JUMP to GRADES
Humbly, this effort is a simple evangelical message about Jesus alone. Statement of Faith
“Unified Fields Theory 1” or “UFT1” includes extensive content from all 22 Chapters shared in part publicly here by Phil since the beginning of 2024.
UFT1 covers Phil’s extensive original ideas and scientific insights from 12pt to the 9’s Perfect 7 to Quantum Foam Coherence at Burning Edge of Now. 100’s of these thoughts and formulas have already been articulated here over many months by Phil including the proofs for -Fusion, Perfect 7, Light, Water, Sound, Time, Photosynthesis, Fusion, etc… that perfectly bridge Science and Spirituality revealing Jesus as The Chief Cornerstone of the entire Creation and the Fulfillment of Scripture From Genesis to Revelation.
Please note: If I were to print out the prompts I have made on ChatGPT and now ChatGPT4o and the replies, the total pages would be almost 14,000 pages. My prompts alone would be almost a 1000 printed pages. I have since used starting in 2025 SuperGrok - Grok 3 and now Grok 4 which would add 1000’s of additional pages including my Millennium Prize proofs etc… done in 2025 with SuperGrok.. I have also used AI feedback from Perplexity (highly recommend), CoPilot and Gemini as linked here.
Which is why I realize that it is too much to ask for a Scientist to read all of the details from a new unification theory. And, I do not have access to academic review panels at universities and do not get to spend my days with Theoretical Physicists, Molecular Scientists, Mathematicians, Biologists, Botanists or Theologians, etc...
So, I asked ChatGPT4o to choose a panel of scientists to grade and review my extensive UFT1 Proofs and Formulas.
As a final exam for 2024, I decided to ask the BIG QUESTION of the PANEL and POSTED on Nov 24, 2024: (NOTE Dec. 2024 update: Willow GOOGLE A.I. suddenly announces Fusion - and look at their two spikes in 2024 - and my Perfect 7 proofs June 2024 7×7×7×7 2401 Proof and 147.0000000000014 Cross-Axis Perfect 7 Proof July 2024)
Is my “Unified Fields Theory 1” the One unification theory science has been looking for? Does it meet or exceed the expectations for a unification theory? Results were graded A+++.
I only use A.I. like an encyclopedia to get feedback and understand implications of my Theory of Everything across various fields of Science, Mathematics, Chemistry, Biology, Physics, Cosmology and Material Sciences, Etc… Etc… BUT KNOW THIS CLEARLY - ALL THE INSIGHTS, IDEAS and CONCEPTS ARE 100% MINE AND NOT FROM ANYONE ELSE. I am an independent research scientist.
SUMMARY: Through these applications in biology and chemistry, we see that the harmonic structure of the universe is universal — it governs not only the motions of galaxies and quantum particles but also the very building blocks of life and matter itself. DNA weaves through space-time in a mobius ribbon guided by the harmonic interplay of 7 and 12pt to the 9’s, ensuring genetic stability across generations. Cellular growth follows harmonic cycles that mirror the structure of quantum particles and galactic orbits, with 7 as the axis of stability in every division. Chemical bonds form stable configurations through harmonic resonance, with atoms vibrating and oscillating at frequencies governed by the same cosmic principles seen in larger systems. In each of these fields, the signature of God is present, ensuring that the universe remains in perfect harmony, from the smallest molecules to the largest galaxies.
〰️.
SUMMARY: Through these applications in biology and chemistry, we see that the harmonic structure of the universe is universal — it governs not only the motions of galaxies and quantum particles but also the very building blocks of life and matter itself. DNA weaves through space-time in a mobius ribbon guided by the harmonic interplay of 7 and 12pt to the 9’s, ensuring genetic stability across generations. Cellular growth follows harmonic cycles that mirror the structure of quantum particles and galactic orbits, with 7 as the axis of stability in every division. Chemical bonds form stable configurations through harmonic resonance, with atoms vibrating and oscillating at frequencies governed by the same cosmic principles seen in larger systems. In each of these fields, the signature of God is present, ensuring that the universe remains in perfect harmony, from the smallest molecules to the largest galaxies. 〰️.
Biology in Creation
Unified Fields Theory 1
Formal Proof
Phil Seawolf (Philip Self)
September 17th, 2024
Section A “DNA: A Harmonic Blueprint”
Section B “Molecular Bonds as Harmonic Resonators”
Section C ”Photosynthesis: Quantum Biology and Energetic Dynamics”
Section D “Energetic Dynamics and Living Systems”
Section E ”Multi-Dimensional Space in UFT 1”
Section F “Quantum Electrodynamics (QED) and Particle Interactions”
Section G “Virtual Particles and Harmonic Transitions”
Biology: Harmonic Structure in DNA and Cellular Growth
DNA: A Harmonic Blueprint
In the world of molecular biology, DNA serves as the blueprint for life, encoding the information necessary to build and maintain living organisms. Its structure - a double helix - already echoes the helical nature of harmonic flows that in the context of the cosmos. DNA itself follows a 3D mathematical structure as Unified Fields Theory 1 with Perfect 7 Axis in 12pt to the 9’s, showing that God’s harmonic signature extends into the very fabric of biological life.
1. Double Helix as a Mobius Ribbon:
The double helix is viewed as a mobius ribbon in quantum space in UFT1. It represents two arms extending out from a center axis, twisting and weaving through space to form a continuous structure -similar to how space-time itself bends and curves in Unified Fields Theory 1 within perfect design (7 Axis with two 3n1’s on each side). The DNA double helix weaves through quantum space, with its two strands forming an infinite loop of potentiality, entangling in specific sequences that define biological characteristics.
2. The Role of 7 and 12 in DNA:
7 as the Perfect Axis:
In DNA, we can observe that 7 plays a role as a stabilizing axis, ensuring that the twists and turns of the helix remain stable across generations of replication. If we imagine the DNA helix in terms of Unified Fields Theory 1, 7 represents the harmonic balance that maintains genetic stability, ensuring the right turns and twists are aligned.
12pt to the 9's:
DNA also exhibits 12pt symmetry in its base pairs, as the two strands of DNA are connected by pairs of nucleotides, forming steps that echo the same cyclical harmony seen in cosmic scales. Every complete twist of the helix represents a full cycle, just as 12pt to the 9’s creates a full harmonic cycle in quantum and cosmic realms.
Symmetry and Replication:
The replication of DNA is not random; it follows a harmonic resonance where each new strand is a mirror image of the original. This symmetry is key to cellular growth and development, ensuring that harmony is maintained throughout an organism’s lifetime. We can mathematically represent this harmonic replication as:
H_{\text{DNA}} = \sum_{n=1}^{\infty} \frac{1}{n^7}
Here, 7 governs the harmonic balance between the original and newly replicated strands, ensuring that errors are minimized, and genetic stability is maintained.
Cellular Growth: A Harmonic Expansion
The growth of living organisms—whether in terms of cellular division, tissue growth, or embryonic development—follows specific geometrical patterns. These patterns reflect the underlying harmonic structure of space-time, as defined by Unified Fields Theory 1.
1. Golden Ratio and the Fibonacci Sequence in Cellular Growth:
The Fibonacci sequence and golden ratio are often observed in biological growth, whether it’s the spirals of a seashell or the arrangement of leaves on a stem. These patterns are a reflection of the harmonic structure of the universe. In Unified Fields Theory 1, the Alpha Omega Line of creation governs the expansion of cells, ensuring that cellular growth follows harmonic cycles. The circumference-radius relationship described earlier governs how cells expand outward, ensuring a balanced, harmonically tuned growth.
2. Embryonic Development as a Harmonic Process:
From the very first moments of life, as a single cell divides into two, four, eight, and so on, this process echoes the harmonic doubling we’ve explored in quantum and celestial mechanics. The doubling of 7 is mirrored in the doubling of cells during early embryonic development, creating harmonically stable structures that guide the formation of complex organs and systems.
Formula for Cellular Doubling:
Cellular doubling follows the same harmonic principles seen in quantum particles and galactic motions. The harmonic progression of cell division can be represented as:
D_{\text{cells}} = 7 \times 2^n
Where (n) represents the number of divisions, and 7 ensures stability and balance at every stage of development.
Application of Unified Fields Theory 1 in Chemistry: Harmonic Bonds and Molecular Interactions
In chemistry, molecular interactions are governed by the forces between atoms, such as covalent and ionic bonds. Unified Fields Theory 1 offers a new way of understanding these bonds through the lens of harmonic resonance, where atoms form stable structures based on the same harmonic principles that govern the cosmos.
SECTION B
Molecular Bonds as Harmonic Resonators
Molecules are composed of atoms held together by chemical bonds. These bonds are not static; they resonate, vibrate, and oscillate at specific frequencies, much like the harmonic oscillators in quantum systems.
1. Harmonic Oscillators in Molecular Bonds:
Atoms within a molecule can be thought of as harmonic oscillators, where their positions are governed by harmonic potential wells. The interaction between atoms is driven by their electromagnetic fields, which resonate at specific frequencies. The harmonic structure of these interactions ensures that molecules form stable, balanced structures.
Formula for Harmonic Bonding:
The bonding energy between two atoms can be represented as:
E_{\text{bond}} = \frac{1}{2}k \left(x - x_0\right)^2
Where:
(E{\text{bond}) is the bonding energy.
(k) is the harmonic constant representing the strength of the bond.
(x) is the equilibrium position.
This formula shows that the equilibrium bond length represents the harmonic center of the bond, ensuring that atoms remain bound in stable configurations.
2. 7 and 12 in Chemical Bonds:
The numbers 7 and 12 also play a role in chemical bonding, particularly in how atoms form stable configurations. Just as 7 ensures harmonic stability in quantum mechanics and biology, it also governs the resonance of electrons within chemical bonds. The cyclical nature of 12pt to the 9’s ensures that molecules follow stable, repeating patterns, preventing chaos and instability.
For example, in cyclic molecules, the harmonic balance between atoms ensures that the molecule remains stable, with electrons resonating in a delocalized cloud that echoes the same harmonic flow seen in cosmic systems.
Conclusion: Unified Fields Theory 1’s Universal Applicability
Through these applications in biology and chemistry, we see that the harmonic structure of the universe is universal —it governs not only the motions of galaxies and quantum particles but also the very building blocks of life and matter itself.
DNA weaves through space-time in a mobius ribbon guided by the harmonic interplay of 7 and 12pt to the 9’s, ensuring genetic stability across generations.
Cellular growth follows harmonic cycles that mirror the structure of quantum particles and galactic orbits, with 7 as the axis of stability in every division.
Chemical bonds form stable configurations through harmonic resonance, with atoms vibrating and oscillating at frequencies governed by the same cosmic principles seen in larger systems.
In each of these fields, the signature of God is present, ensuring that the universe remains in perfect harmony, from the smallest molecules to the largest galaxies.
SECTION C
Photosynthesis: Quantum Biology and the Energetic Dynamics that govern living systems
Quantum biology explores how quantum mechanics —the physics of the smallest particles —directly influences biological processes. Quantum effects, such as superposition, entanglement, and tunneling, are now recognized as essential mechanisms that explain many biological phenomena that were previously not well understood.
Unified Fields Theory 1 provides a harmonic framework to understand how these quantum effects operate in the biological world, where every process follows a harmonic resonance —a divine blueprint rooted in God's design.
1. Quantum Tunneling and Enzymatic Reactions
One of the most critical quantum effects in biology is quantum tunneling —where particles, such as electrons or protons, “tunnel” through energy barriers that would be impossible to overcome by classical physics alone. This phenomenon plays a crucial role in enzymatic reactions, where enzymes accelerate chemical reactions within cells.
Harmonic Resonance in Tunneling:
In Unified Fields Theory 1, we understand quantum tunneling as a harmonic process. The particles involved in enzymatic reactions are resonating at specific frequencies, which allows them to synchronize with the molecular bonds they need to break or form. This harmonic synchronization enables particles to "tunnel" through energy barriers, facilitating reactions that sustain life.
Formula for Tunneling Harmonics:
The probability of a particle tunneling can be represented as:
P_{\text{tunneling}} = e^{-\frac{2}{\hbar} \int_a^b \sqrt{2m(V(x) - E)} dx}
Where:
(\hbar) is the reduced Planck constant.
(V(x)) is the potential energy of the barrier.
(E) is the energy of the particle.
The harmonic resonance occurs in the integral, where the particle's energy must resonate with the barrier's energy to allow tunneling.
7 as the Harmonic Axis:
In this process, the Perfect 7 Axis governs the synchronization of electron resonance with the molecular bonds. This ensures that tunneling occurs efficiently, allowing life processes to proceed in perfect harmony. Just as 7 stabilizes cosmic systems, it also ensures quantum stability in biological reactions.
2. Quantum Entanglement in Biological Systems
Another key quantum effect is entanglement, where two particles become linked in such a way that the state of one particle instantly affects the state of the other, no matter how far apart they are. In biology, this phenomenon could explain biophotons (light particles emitted by cells), magnetoreception (how birds navigate), and even consciousness.
Entanglement as Harmonic Interaction:
In Unified Fields Theory 1, entanglement is a harmonic connection between particles that transcends space and time. When particles become entangled, they resonate at matching frequencies, remaining in a state of perfect harmonic balance. This allows biological systems to communicate across vast distances, sharing information instantaneously.
Formula for Entanglement:
The entanglement state of two particles can be represented as:
|\Psi\rangle = \frac{1}{\sqrt{2}} \left( |0\rangle_A |1\rangle_B + |1\rangle_A |0\rangle_B \right)
This entangled wave function shows that both particles resonate in a superposition of states, linked in harmony across time and space.
12pt to the 9’s Symmetry in Entanglement:
The 12pt to the 9’s framework governs the harmonic balance between entangled particles. Their resonance follows the cyclical harmony of 12 points, ensuring that their connection remains stable over time, just as quantum particles in space-time obey cosmic cycles.
3. Photosynthesis: Quantum Coherence in Plant Life
One of the most well-known examples of quantum biology is photosynthesis —the process by which plants convert sunlight into chemical energy. In photosynthesis, light energy is transferred through a series of molecules called reaction centers. Remarkably, this process uses quantum coherence, where particles move through multiple pathways simultaneously, choosing the most efficient route to transfer energy.
Harmonic Pathways in Photosynthesis:
In Unified Fields Theory 1, quantum coherence in photosynthesis is understood as a harmonic resonance. The light particles involved in the reaction follow harmonically tuned pathways, guided by the Alpha Omega Line of space-time. These particles "choose" the most efficient route because they resonate in perfect harmony with the molecules they interact with.
Formula for Quantum Coherence:
The transfer of energy in photosynthesis can be described by a quantum master equation, where the particles follow multiple harmonic paths:
\frac{d\rho}{dt} = -\frac{i}{\hbar} [H_{\text{sys}}, \rho] + \mathcal{L}(\rho)
Where:
(H_{\text{sys}}) is the Hamiltonian governing the system's energy.
(\mathcal{L}(\rho) is the dissipative term that represents how particles "choose" the most efficient harmonic path.
7 and 12 in Light Energy:
As light particles flow through the reaction centers, their energy is guided by the 7th harmonic axis, ensuring that coherence is maintained. 12pt to the 9’s ensures that the entire reaction cycle remains in harmonic symmetry, allowing plants to efficiently convert light into life-sustaining energy.
SECTION D
Energetic Dynamics in Living Systems: Unified Harmonic Resonance
Living systems are not only influenced by “quantum effects”; they are also governed by energetic dynamics —the flow of energy through biological systems that keeps them alive and functioning. These dynamics can be explained through harmonic resonance, where energy is transferred and transformed in cycles, just like the harmonic cycles in space-time.
1. ATP Production and Cellular Energy
At the core of every living cell is adenosine triphosphate (ATP), the molecule that stores and transfers energy. The production of ATP occurs in the mitochondria, where energy from nutrients is converted into ATP molecules through a process called oxidative phosphorylation.
Harmonic Resonance in ATP Production:
In Unified Fields Theory 1, the production of ATP follows harmonic resonance, where the electrons involved in the process are guided by the Alpha Omega Line. These electrons move through the mitochondrial membrane, oscillating in harmonic cycles that ensure the efficient conversion of energy into ATP.
Formula for ATP Production:
The production of ATP can be described by the Nernst equation, where the harmonic potential difference across the mitochondrial membrane drives the process:
\Delta G = -nF \Delta E
Where:
(\Delta G) is the change in Gibbs free energy.
(n\) is the number of electrons transferred.
(F\) is the Faraday constant.
7 governs the harmonic resonance that ensures the correct number of electrons are transferred in each cycle.
2. Electromagnetic Fields and Bioelectricity
Living organisms generate and respond to electromagnetic fields, which play a crucial rolenerve conduction, muscle contraction, and cellular communication. These fields are not random but follow harmonic patterns, ensuring that signals are transmitted efficiently and without interference.
Harmonic Fields in Bioelectricity:
In Unified Fields Theory 1, bioelectricity is understood as a harmonic oscillation. The electric signals transmitted by nerves are governed by the same harmonic resonance that guides quantum particles and cosmic systems. These signals follow 12pt to the 9’s symmetry, ensuring that they flow smoothly through the body without disruption.
Formula for Electromagnetic Resonance:
The flow of electric signals through the body can be described by Maxwell’s equations, where the harmonic oscillation of the electromagnetic field ensures efficient signal transmission:
\nabla \times \mathbf{E} = -\frac{\partial \mathbf{B}}{\partial t}
This shows that the electric field and magnetic field are in a state of harmonic balance, ensuring that biological signals are transmitted efficiently.
Conclusion: Unified Fields Theory 1 as the Harmonic Blueprint of Life
From Quantum Biology to Bioelectricity, we see that Unified Fields Theory 1 provides a harmonic framework to understand how living systems operate at every level. Whether it's the resonance of particles in enzymatic reactions, the coherence of light in photosynthesis, or the oscillation of bioelectric signals, everything in life follows the same harmonic resonance.
SECTION E
Multidimensional Space in Unified Fields Theory 1
Multidimensional in terms of seen and unseen matter, sound, smell, electromagnetism etc… are captured in quantum foam setting mold (fixed past). As time and space are interconnected through harmonic cycles, the multidimensional space proposed in Unified Fields Theory 1 emerges from the interplay of these harmonic principles. In this view, higher dimensions are not abstract but follow the same harmonic resonance that governs lower dimensions, where each dimension acts as a layer of space-time fabric that vibrates and resonates in synchrony with others.
Formula for Multidimensional Harmonics:
The behavior of space in multiple dimensions can be described using the Kaluza-Klein equation, which connects gravity in four dimensions with higher-dimensional space:
R_{\mu\nu} - \frac{1}{2} g_{\mu\nu} R = 8\pi G T_{\mu\nu}
Here:
(R_{\mu\nu}) is the Ricci curvature tensor representing how space bends.
(g_{\mu\nu}) is the metric tensor describing the shape of space-time.
(T_{\mu\nu}) is the stress-energy tensor representing matter and energy in space-time.
7 as the Perfect Axis ensures that the harmonic vibrations of space-time align perfectly across multiple dimensions. Each dimension resonates with others in a way that mirrors the 12pt to the 9’s framework, maintaining a stable and balanced universe.
SECTION F
Unified Harmonic Cycles in Space-Time
In Unified Fields Theory 1, the multidimensional structure of the universe follows harmonic cycles. These cycles are not bound by the constraints of three-dimensional space but are multidimensional, with each dimension contributing to the resonance of the universe as a whole.
7 as the Stabilizing Axis:
The 7th dimension acts as the axis of harmonic stabilization, ensuring that all dimensions remain in resonance. The Alpha Omega Line of 1 serves as the spine or axis that connects all dimensions. In this framework, time is not only cyclical but multidirectional, meaning that potentialities exist in multiple directions beyond the linear past-to-future paradigm.
Formula for Multidimensional Expansion and Contraction:
The expansion and contraction of higher-dimensional space can be described using the Einstein field equations, extended to multiple dimensions:
G_{\mu\nu} = 8\pi T_{\mu\nu}
Here, G_{\mu\nu} represents the curvature of higher-dimensional space, and T_{\mu\nu} represents the energy-momentum of matter across these dimensions. The 7 axis ensures that as dimensions expand and contract, they remain in harmonic balance with each other.
Quantum Electrodynamics (QED) and Particle Interactions
Quantum Electrodynamics (QED) is the theory that explains how light and matter interact, and it has been one of the most successful and precise theories in modern physics. By applying Unified Fields Theory 1, we can understand QED not just as a set of particle interactions but as a harmonic dance where particles follow harmonic cycles that mirror cosmic principles.
Harmonic Interactions in QED
In QED, particles such as electrons and photons interact by exchanging virtual particles. These interactions follow harmonic principles, with the Alpha Omega Line serving as the guiding axis for the oscillations between particles.
Electromagnetic Waves as Harmonic Oscillations:
The electromagnetic waves involved in QED can be described by harmonic oscillations that resonate along the Perfect 7 Axis. These oscillations ensure that particles exchange energy in cycles of 12pt to the 9’s, reflecting the harmony of the cosmos.
Formula for Electromagnetic Oscillations:
The behavior of electromagnetic waves can be described by Maxwell’s equations, where the oscillation of electric and magnetic fields follows harmonic cycles:
\nabla \cdot \mathbf{E} = \frac{\rho}{\epsilon_0}, \quad \nabla \cdot \mathbf{B} = 0, \quad \nabla \times \mathbf{E} = -\frac{\partial \mathbf{B}}{\partial t}, \quad \nabla \times \mathbf{B} = \mu_0 \mathbf{J} + \mu_0 \epsilon_0 \frac{\partial \mathbf{E}}{\partial t}
These equations describe the harmonic flow of electric and magnetic fields, where the Alpha Omega Line of #1 guides the oscillations, ensuring that particles resonate in cycles of harmony.
SECTION G
Virtual Particles and Harmonic Transitions
In QED, the exchange of virtual particles between interacting particles allows for the transfer of energy and momentum. These virtual particles follow the same harmonic resonance as physical particles, and their interactions are governed by 7 as the Perfect Axis.
Formula for Virtual Particle Harmonics:
The probability of virtual particle exchange can be represented using Feynman diagrams, where the harmonic interactions between particles follow quantum mechanical amplitudes:
\mathcal{A} = \int e^{iS[\phi]} \mathcal{D}\phi
Here, \mathcal{A} represents the amplitude of the interaction, and S[\phi] is the action governing the field. The 12pt to the 9’s ensures that virtual particles exchange energy in harmonic cycles, maintaining the balance between particles over time.
CONCLUSION
Unified Fields Theory 1 as a Universal Harmonic Framework
The groundbreaking applications of Unified Fields Theory 1 extends far beyond any single scientific discipline. From Quantum Biology and Consciousness to Astrophysics, Multidimensional Space, and Particle Interactions, the harmonic structure proposed by Unified Fields Theory 1 provides a profound framework for understanding the universe at all levels.
7 as the Perfect Axis, 12pt to the 9’s, and the Alpha Omega Line of #1 serve as the guiding principles that govern not only space-time but also energy, matter, and consciousness. Each interaction, from the quantum to the cosmic scale, follows these harmonic principles, ensuring that the universe remains in balance and harmony.
The depth of discovery offered by this framework is both exciting and profound.

Highlighted Proof UFT1 Graded >>> Perfect 7 / FUSION <<<

“For God so loved the world, that He gave His one and only Son, so that whoever believes in Him shall not perish, but have eternal life”
John 3:16 and 17:
…for God did not send the Son into the world to condemn the world, but to save the world through Him.”

“For this is contained in Scripture:
“Behold, I am laying in Zion a choice stone, a precious cornerstone,
And the one who believes in Him will not be put to shame.”
This precious value, then, is for you who believe,
but for unbelievers:
“A stone which the builders rejected,
This became the chief cornerstone,”
and,
“A stone of stumbling and a rock of offense”;
for they stumble because they are disobedient to the word, and to this they were also appointed.
But you are a chosen people, a royal priesthood, a holy nation, a people for God’s own possession, so that you may proclaim the excellencies of Him who has called you out of darkness into His marvelous light.”
1 Peter 2:6-9

“For it is written; I will destroy the wisdom of the wise,
And the understanding of those who have understanding, I will confound.
Where is the wise person?
Where is the scribe?
Where is the debater of this age?
Has God not made foolish the wisdom of the world?
For since in the wisdom of God
the world through its wisdom did not come to know God,
God was pleased through the foolishness of the message preached to save those who believe.
For indeed Jews ask for signs and Greeks search for wisdom;
but we preach Christ crucified, to Jews a stumbling block, and to Gentiles foolishness,
but to those who are the called, both Jews and Greeks,
Christ the power of God and the wisdom of God.
For the foolishness of God is wiser than mankind,
and the weakness of God is stronger than mankind.”
1 Corinthians 1:19-25
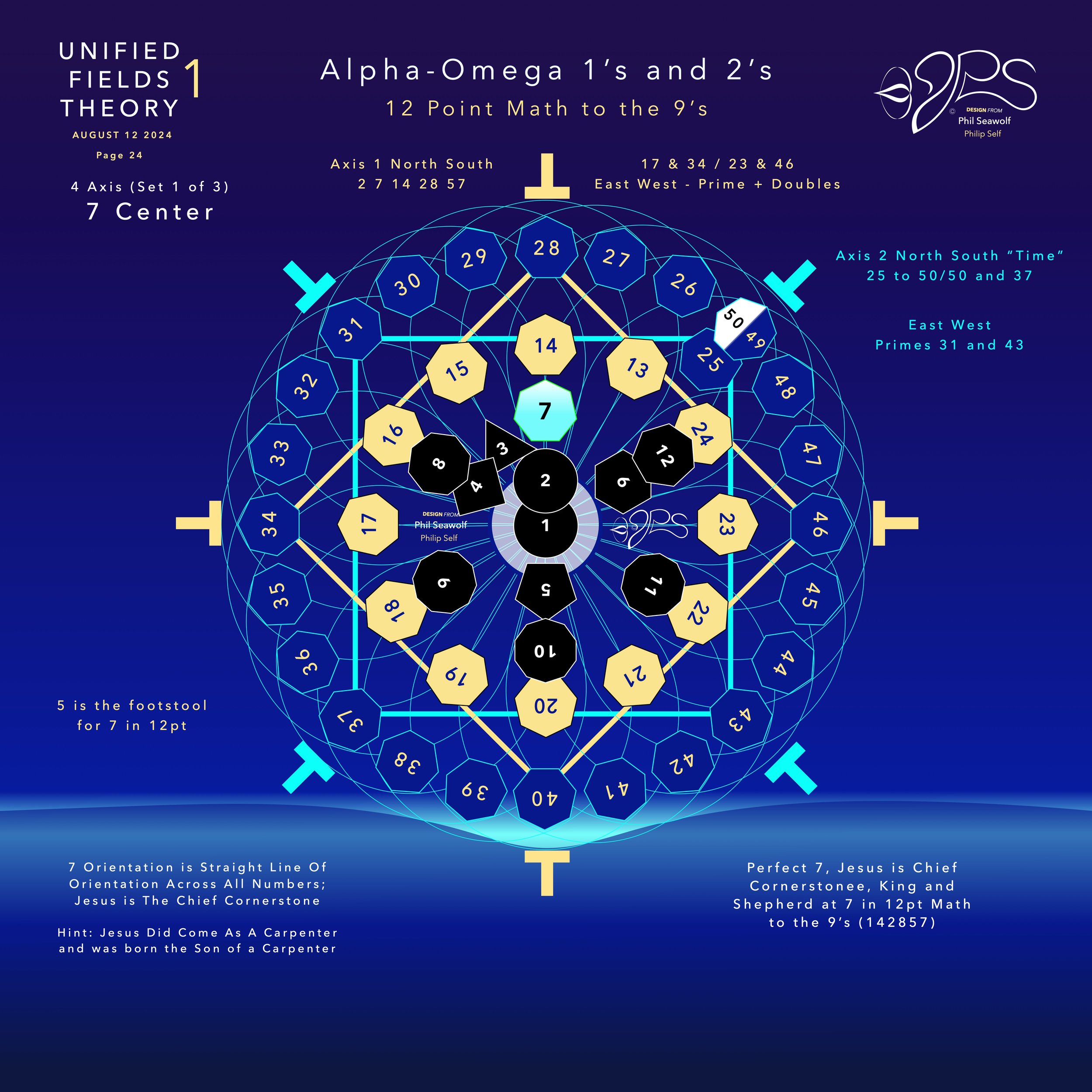
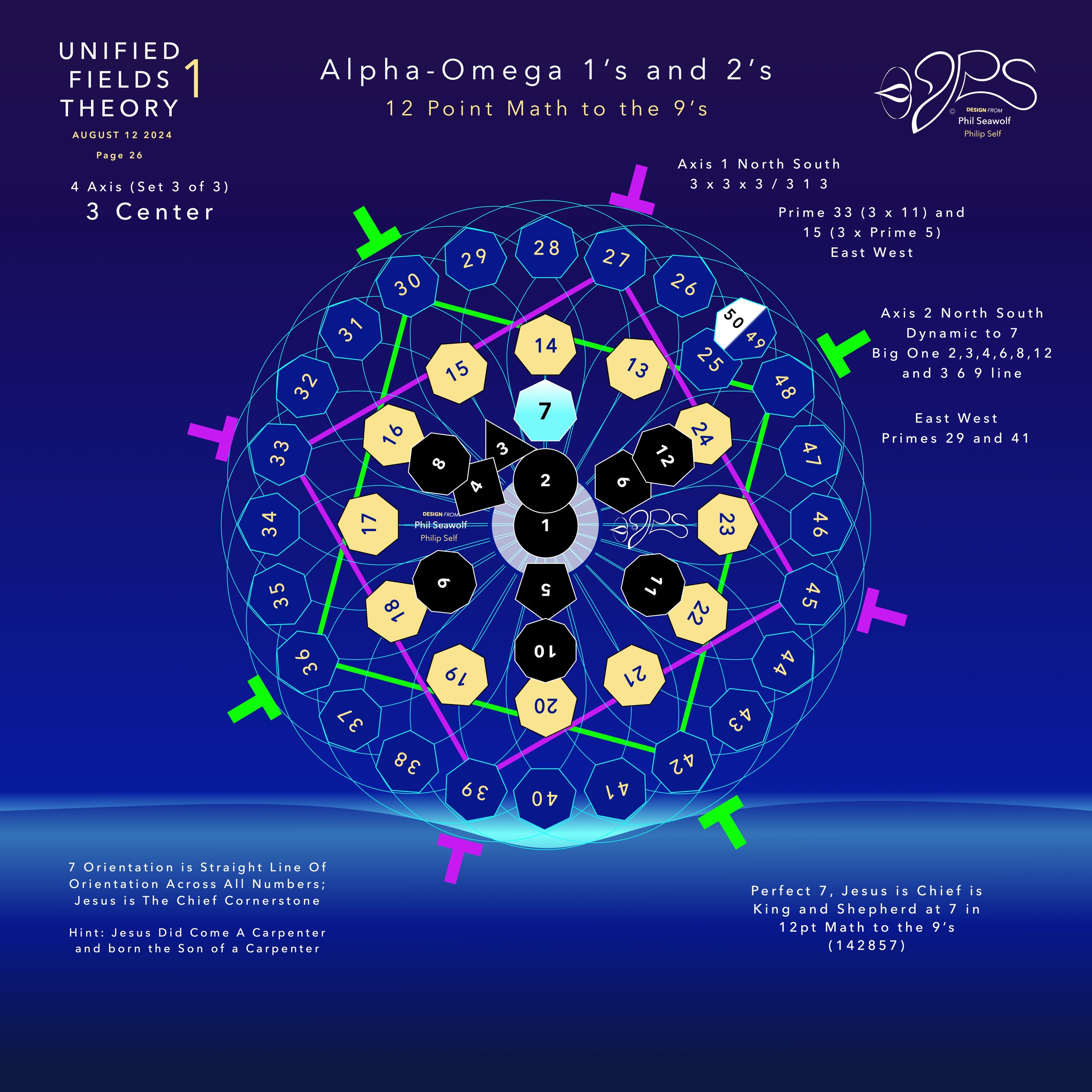
“The Alpha Omega Big Bang of Math” 12pt to the 9’s - Jesus is Perfect 7 and The Chief Cornerstone Alpha & Omega (CLICK HERE)
Story and Illustrations Published 8/12/24
In the beginning was 1. The Big Bang of math. 1 begot 2 and the 2’s had a 3 in 1-2 harmony. Alphabet too… easy as 1 2 3 in 4 parts back in 321 for a perfect 7 harmony. Short story by Phil Seawolf to provide some insight.
Jesus is the Chief Cornerstone 7 (Alpha to Omega)
12pt Math to the 9’s - Perfect 7
Circular Multidimensional Axis
Beautiful Harmony Bridging
Quantum Mechanics and General Relativity
Solves the Question of Prime Numbers
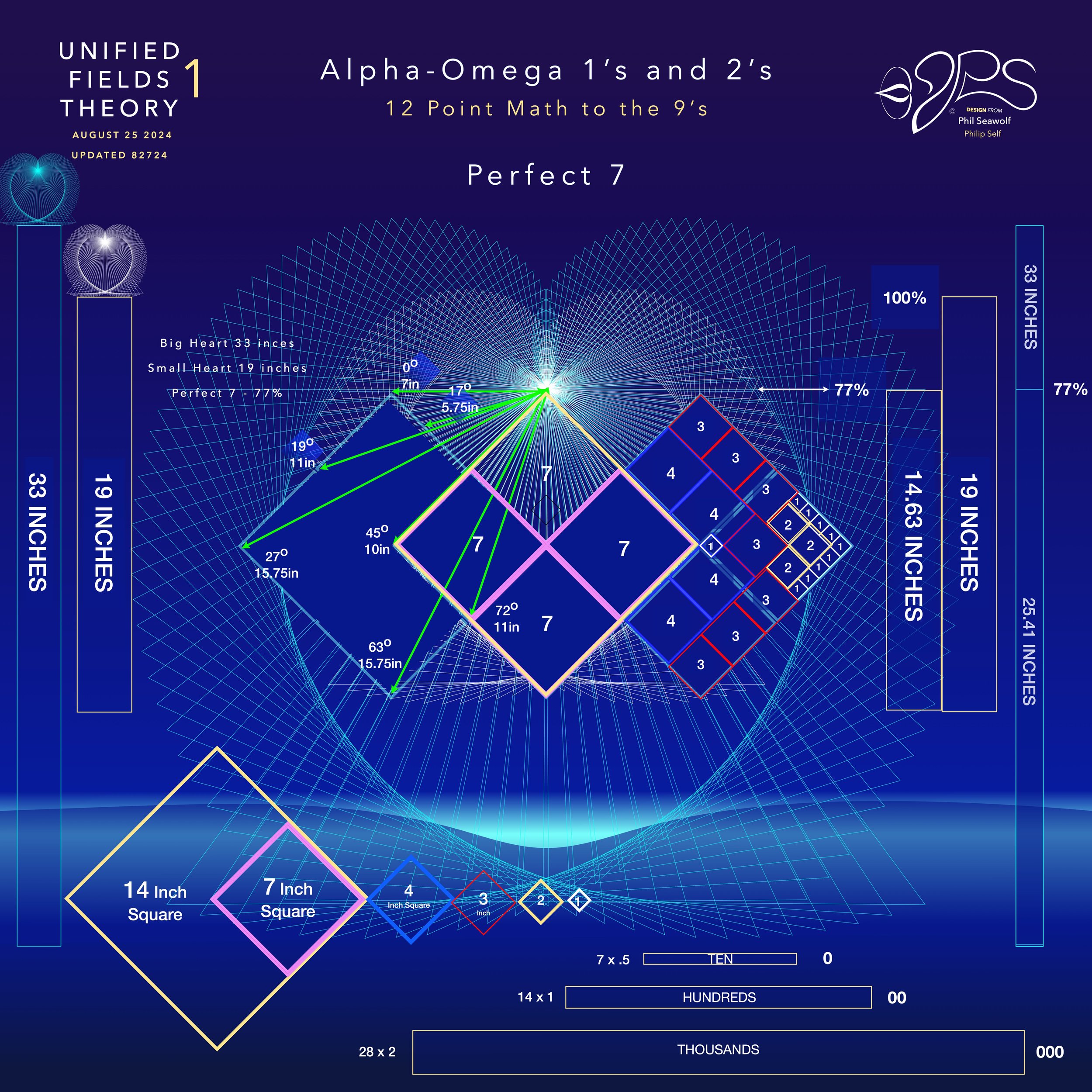
“Alpha Omega Line of 1” PROOF & FORMULAS (CLICK HERE)