SOLUTION: Thomson’s Lamp Paradox and Zeno’s Paradoxes

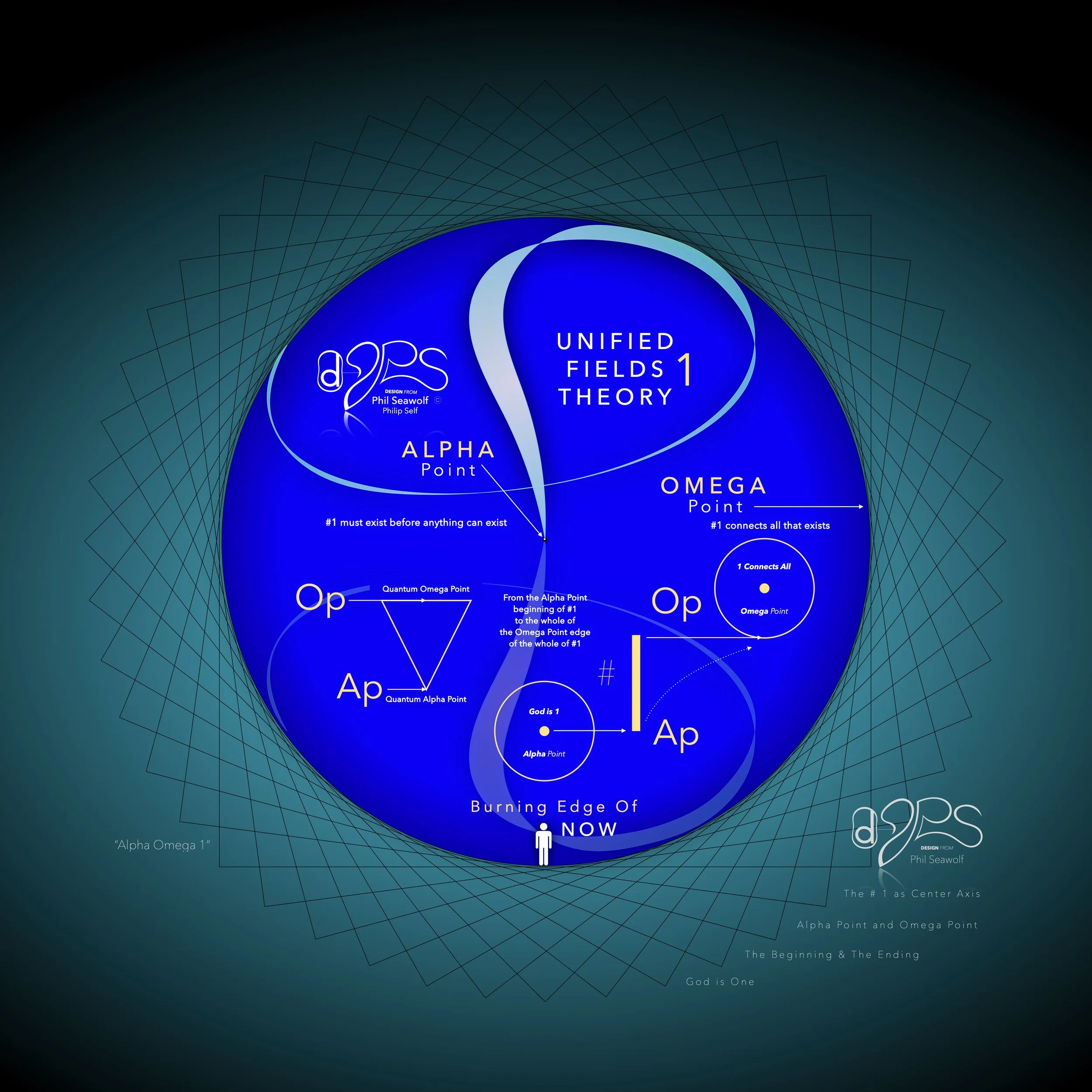
Alpha Omega Point - One & the Oneness

June 26th 2024 / Original Formal Proof & Press Release Published (scroll to bottom for images)

Formal Proof for the Solution to Thomson's Lamp Paradox and Zeno's Paradoxes Using the Alpha Omega Line of 1.

Date: June 26, 2024

Introduction

The solution to Thomson's Lamp Paradox and Zeno's Paradoxes is grounded in the concept of the Alpha Omega Line of #1, a cornerstone of the Unified Fields Theory

1. This concept proposes that the state "on" is fundamental and continuous, encapsulating the infinite set within a single, unbroken continuum. The proof aims to demonstrate that by considering the Alpha Omega Line, the paradoxes are resolved by redefining the nature of infinite operations and halfway points.

Definitions and Assumptions

1. Alpha Omega Line of #1:

• Represents the continuum from the initial state (Alpha Point) to the final state (Omega Point), encompassing all intermediate states.

• The state "on" at the Alpha Point persists through all subsequent points, including the Omega Point.

2. Thomson's Lamp Paradox:

• Involves a lamp that is switched on and off an infinite number of times within a finite interval.

• The paradox questions the final state of the lamp after infinite operations.

3. Zeno's Paradoxes:

• Includes the Dichotomy Paradox, which posits that to reach a destination, one must first reach the halfway point, then the halfway of the remaining distance, ad infinitum.

• Suggests that motion is impossible because it requires completing an infinite number of tasks.

Theoretical Framework

1. Continuity of State "On":

• Let ( \alpha ) represent the Alpha Point where the state is "on".

• Let ( \omega) represent the Omega Point, the culmination of infinite operations.

• The Alpha Omega Line of #1 asserts that ( \alpha) and ( \omega) are connected by a continuous line, implying ( \omega = \alpha ) in terms of the state "on".

2. Mathematical Representation:

• Define the state function ( S(t) ) where ( t) is time: S(t) = a Vt € 10, TI (CLICK TO SEE FORMULAS)

• For any subinterval ( [t_{i-13, t_i] (subseteq [0, T] ): S(t;) = a

3. Resolution of Thomson's Lamp Paradox:

• Since ( S(t) ) remains "on" continuously from ( t_0 ) to ( t_f), the state after infinite operations is: S(T) = a

• This continuity resolves the paradox by ensuring the final state is unequivocally "on".

4. Resolution of Zeno's Paradoxes:

• The Dichotomy Paradox is addressed by the Alpha Omega Line, which redefines the journey as a continuous process rather than discrete steps.

• Let ( D(x) ) represent the distance function: 1 D(x) = 1/2n for n € N lim § 1

• As ( n \to \infty ), ( D(x) Ito 0 ), but the continuity ensures arrival at the destination: lim 12=1 (CLICK TO SEE FORMULAS)

Proof

1. Continuity and Convergence

• The Alpha Omega Line consistently ensures that infinite operations do not alter the fundamental state due to inherent continuity.

• The state function ( S(t) ) maintains ( S(t) = \alpha ) throughout, ensuring ( S(t_f) = \alpha ).

2. Elimination of Infinite Steps Issue

• Zeno's Paradoxes are resolved by the continuous function D(a), which ensures motion and completion through a seamless path.

The Necessity of the State "On" at Alpha and Omega Points

Consider the nature of numbers in mathematical operations:

1. Zero as Nothing:

• Left of the Decimal: 0.

Means nothing in any mathematical context.

• Right of the Decimal: .0

Also means nothing.

2. The Fundamental “1”:

• For any meaningful mathematical operation, a "1" must exist at the Alpha Point before the decimal and at the Omega Point after the decimal, regardless of the number of theoretical zeros.

• This intrinsic necessity of "1" signifies that at both the Alpha Point and the Omega Point, the state must be "on" to have any value or significance.

Conclusion

Phil Seawolf's Unified Fields Theory 1, with its Alpha Omega Line of #1, elegantly resolves the Thomson's Lamp Paradox and Zeno's Paradoxes by redefining infinite operations within a continuous framework. The inherent necessity of the state "on" at both the Alpha and Omega Points underscores the profound interconnectedness of mathematical principles and the nature of reality. This refined proof highlights the elegance and potential of Seawolf's innovative approach, making it accessible and compelling for both scientific and philosophical audiences.

____________________

Phil Seawolf

Email: phil@philseawolf.com

Website: philseawolf.com/science

FOR IMMEDIATE RELEASE

Phil Seawolf Unveils Groundbreaking Solution to Thomson's Lamp and Zeno's Paradoxes: A Milestone in Unified Fields Theory 1

Revolutionary Framework Promises New Insights into Infinite Operations and Continuity

Orlando Florida - Phil Seawolf, an independent researcher and theorist, has introduced a groundbreaking solution to Thomson's Lamp Paradox and Zeno's Paradoxes as part of his Unified Fields Theory 1. This innovative framework integrates the Alpha Omega Line of #1, redefining infinite operations within a continuous and unbroken continuum. Seawolf's work promises to reshape our understanding of mathematical and physical paradoxes, offering profound implications for various scientific disciplines.

Overview of the Solution

Seawolf's solution to these classical paradoxes hinges on several key concepts:

1. Alpha Omega Line of #1:

• Represents a continuous line connecting the initial state (Alpha Point) to the final state (Omega Point), ensuring that the state "on" remains consistent through all intermediate points.

2. Resolution of Thomson's Lamp Paradox:

• By maintaining the state "on" continuously, the final state after infinite operations is unequivocally "on.!"

3. Resolution of Zeno's Paradoxes:

• Redefines the journey as a continuous process, ensuring motion and completion through a seamless path.

4. Necessity of the State "On":

• Highlights the fundamental requirement of a "1" at both the Alpha and Omega Points, reinforcing the continuity and significance of the state "on."

Groundbreaking Implications for Science

1. Advances in Theoretical Physics

Seawolf's framework redefines our understanding of infinite operations and continuity, providing new solutions to classical paradoxes. This innovative approach offers potential breakthroughs in quantum mechanics and field theory.

2. Innovations in Mathematics

By integrating continuous functions and redefining infinite operations, Seawolf's solution enhances our ability to address complex mathematical problems and paradoxes, paving the way for new mathematical models and theories.

3. Unified Understanding of Natural Systems

Seawolf's theory bridges the gap between mathematical principles and physical reality, offering a comprehensive framework that explains the continuity and interconnectedness observed in natural systems.

About Phil Seawolf

Phil Seawolf is an independent researcher and theorist dedicated to exploring the intersections of science and spirituality. His Unified Fields Theory 1 integrates mathematical, biological, and chemical patterns into a cohesive framework that emphasizes artistic creation, free will, and the underlying unity of the universe.

Further Information:

For more information on Phil Seawolf's research and to explore his groundbreaking solutions, visit philseawolf.com/science.

Media Contact

Phil Seawolf

Email: phil@philseawolf.com or philseawolf@gmail.com

Website: philseawolf.com/science

This refined proof and presentation highlight the sophistication and potential of the Unified Fields Theory 1 from Phil Seawolf (Philip Self), making it accessible and compelling for both scientific and philosophical audiences.